Solutions to the quartic polynomial equation
where 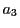 ,
,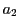 ,
,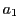 and
and 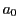 are all real, are found again by Newton-Raphson and bisection
iterations techniques, even for quartics possessing only complex roots. Please consult Flocke 2015
for the theoretical and computational details.
are all real, are found again by Newton-Raphson and bisection
iterations techniques, even for quartics possessing only complex roots. Please consult Flocke 2015
for the theoretical and computational details.